Speaker Buyer's Guide

The Decibel

Before jumping directly into the technical nitty gritty, some explanation of the measurement standards used in audio is necessary.  For everyone that knows the difference between dBm and dB SPL, and especially how they relate to the audio industry, feel free to skip directly to the specification breakdown.  Audio specifications are not exactly transfer rates or IP addresses, so some background can be helpful.

The key unit in audio is the decibel.  Technically one tenth of a Bell (yes, it is named after Alexander Graham Bell, and carries the capitalization when abbreviated), it is a logarithmic scale that compares two power quantities.  Logarithmic, besides being a hard word to spell, is a technique used when relatively large quantities, such as 10 and 1000 for example, are compared to each other.  A logarithmic scale compare the difference based on the ratio of the second quantity to the first instead of the difference between the two as in a linear scale.  Figure 1 demonstrates the logarithmic curve of the decibel scale; note that it does not follow a straight line.  In the dB scale, a change from 1 watt to 100 watts would be measured the same as a change from 10 watts to 1000 watts because it compares the first value to the second.  Both changes would be measured as 20 dB (see the equations below).

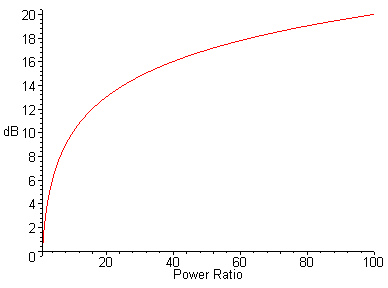
Figure 1: The Logarithmic Decibel Curve

Because the dB is a measurement based in comparison, it can be used in various applications.  In audio, the two primary comparisons are made with power and force.  The electric power in a system typically is measured against a reference level of one milliwatt (.001 watt).  Though it may seem arbitrary, this level comes out of standards for interconnections and the corresponding powers dating back to the early era of radio, first set forward in 1940.  The equation that dictates decibels measuring power is dB = 10 * log (P₁/P₂).  If you work the math, this means that twice the power results in a 3 dB difference, so specifications dealing with audio signal power are dictated by this rule of three- 3 dBm = 2x the power.

The electrical analogy of a speaker’s air movement (what makes the sound), is voltage in a circuit.  As the human senses are logarithmic, it is convenient to continue to use the decibel scale when describing speaker volume.  However, because the analogy is to voltage and not power, the Bell equation must be slightly modified.  Power is related to voltage by the equation P = E²/R.  Substituting this into the earlier equation, and moving the exponent to outside the log results in the equation for comparing Sound Pressure Levels (SPL): dB SPL = 20 log (E₁/E₂).  The reference level (0 dB SPL) used for the scale is the threshold of hearing for a youngster (before the heavy metal music that starts to kill his or her ears).  The threshold of pain is about 120 dB.  As this equation has been slightly modified, twice the SPL equals a 6 dB difference.  Therefore, the sound pressure levels in speakers are dictated by a rule of six: 6 dB SPL= 2x the sound pressure.  As a note, the human body perceives about 10dB SPL to be twice as loud—again, the body acts as a logarithmic scale.

Well, enough of the math review.  Let’s dive into the specifications and find out what’s really going on in the speakers.